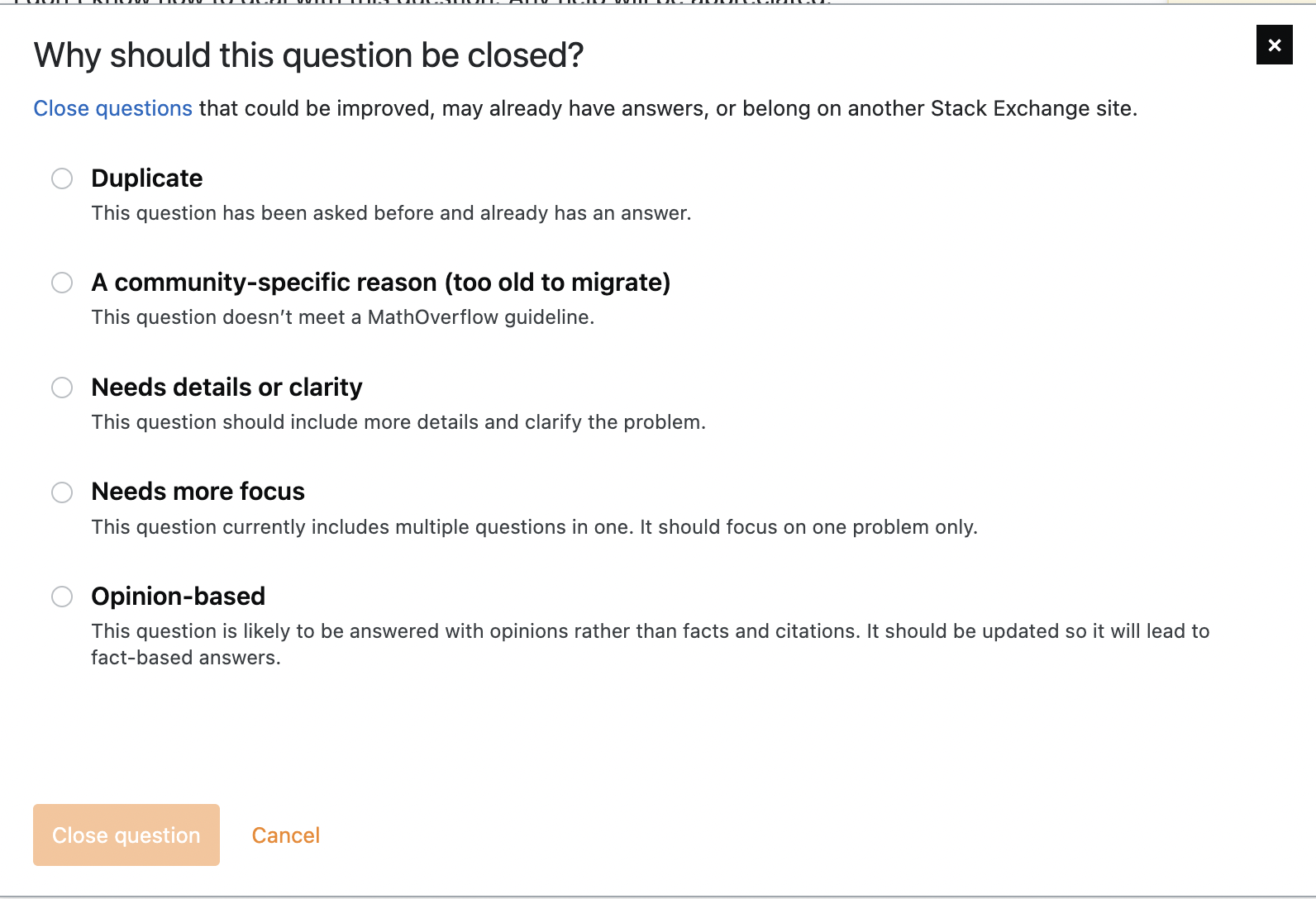
I wanted to vote to close this question which claims to disprove the Riemann Hypothesis, but I realized that, even though we get questions of this kind occasionally, none of the reasons to close is really appropriate. It's not “duplicate”, doesn't need “details or clarity”, doesn't “need more focus”, isn't “opinion-based”, doesn't belong in a different StackExchange network, and it doesn't seem right to add an ad hoc comment (except if we had a clear FAQ to link to, see last paragraph). We could make the case that it's “not about research level mathematics”, but I think this somewhat misses the point (RH is clearly research-level mathematics).
Here and here are other Meta questions about this kind of issue, and while there is clear consensus that such questions need to be closed or deleted, there doesn't seem to be much agreement as to how to do it.
Would it be possible to add another reason for closure that could look something like this: “MathOverflow is not a place to publish claims of proofs or discuss whether such proofs are correct”?
Also (and I realize this is a somewhat different question), is there a standardized FAQ to which to redirect people who claim to have solved this-or-that famous problem, that explains (politely but firmly) why experts can't afford to take the time to read such claims? Because the typical answer of “send it to a journal” really isn't honest: no journal is going to even attempt to referee a one-page paper (or a 1000-page paper, for that matter) coming from J. Random Nobody with claims to prove or disprove RH, it's just going straight to the garbage can, and the note would even be rejected from the arXiv. (We can tell them to post them on viXra, but it would be a lie to say that any expert would ever read them.) A more honest answer would be something like “if you can prove or disprove RH, then you can probably start by making a name for yourself by tackling on some other easier problems”, but probably someone wrote such an explanation down somewhere already and we can just link to it.