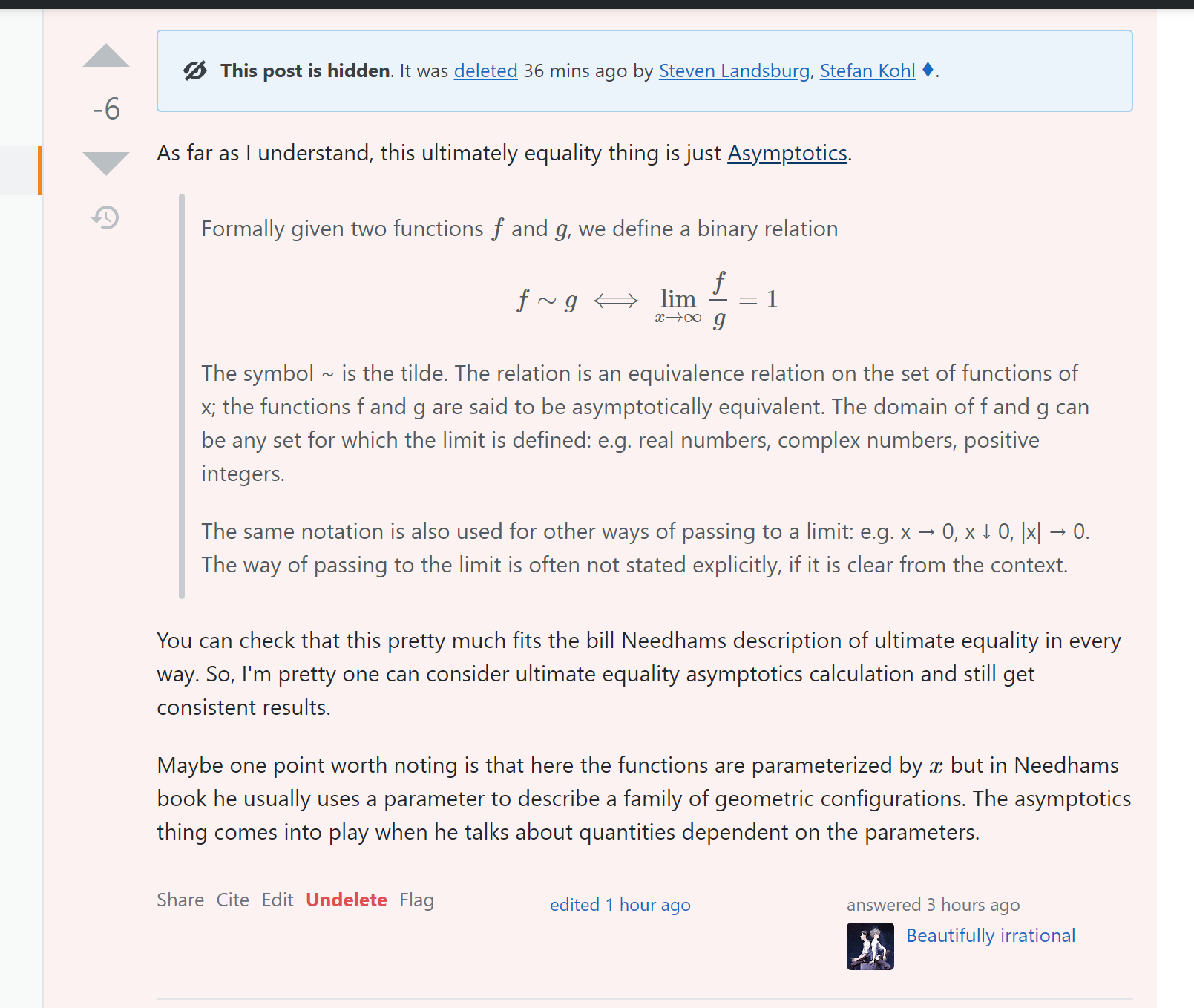
I had recently posted this answer which got 3 dvs plus a delete vote shortly after posting. I was quite upset by this because I was fairly confident with my answer as I actually read a considerable chunk of both of Needhams book.
If there is a mistake in my judgement, could it be that the mistake is pointed out instead of giving a barrage of dvs? Thanks.
Here is a picture of the post:
One person commented how it is that I am identifying asymptotics is the ultimate equality. It simply is the same thing. I believe Needham himself invented the equality for the book as I have gone through many books and have yet to find a similar concept being used to describe the issue.
Needham himself states in this book that the concept is more or less "proof beyond reasonable doubt" in the common sense than a proof in a rigorous mathematical sense
Further to verify my claims, Needham himself mentions that the ultimate equality is an equivalence relation:
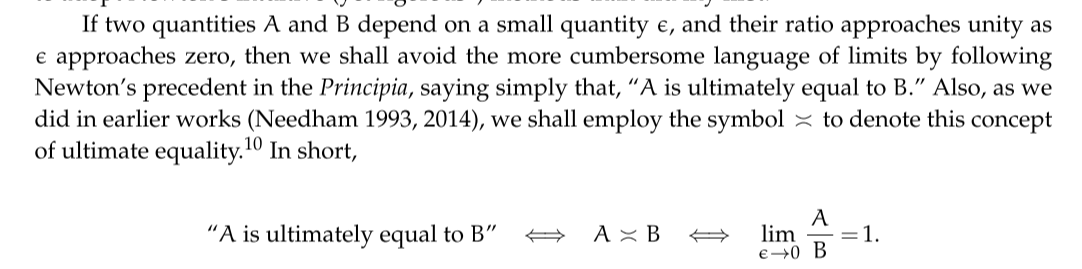
If an exact definition of this concept is sought, I doubt that any possible person can give an authoritative answer to it. As only Needham would know as this concept with the name that Needham used is not shown in any standard DG books.
Edit:
I have included this section in hopes of showing how my answer solves the question.