Long display style formulae extend too much to the right with some renderers

Question

In the following screen capture, take a look at the region delimited by the red rectangle: notice how it extends too much to the right, such that a part of it becomes hidden by the yellow page element.

When editing the question (which in particular allows you to see that it is indeed a huge display style formula) with the preview feature enabled, the same red rectangle shows below how this formula extends uncontrollably to the right.

This happens with the most recent versions of both Firefox and MS Edge, so it is not a browser problem. It is also not a new problem, I remember having encountered it in the past but never having bothered to report it. (It could also affect MSE.)

The only renderer that is affected by this bug seems to be Common HTML. The other rendereres use different fonts which make the whole line narrower and less needy for space at the right, with the exception of the HTML CSS renderer which uses the same fonts as Common HTML but is smart enough to break that formula across two lines.

Answer 1

Perhaps we might create here some examples which illustrate the bug. (So that it is not necessary to rely on a link to a post on the main site.)

Whether the bug manifests or not might depend on many various aspects, but some longish centered formulas should probably suffice to illustrate the problem. (Most likely they will be a bit artificial, but that doesn't really matter that much if the purpose is to illustrate behavior of some specific MathJax renderer.)

This answer is community wiki - do not hesitate to edit it further, if you have suitable additions.

---

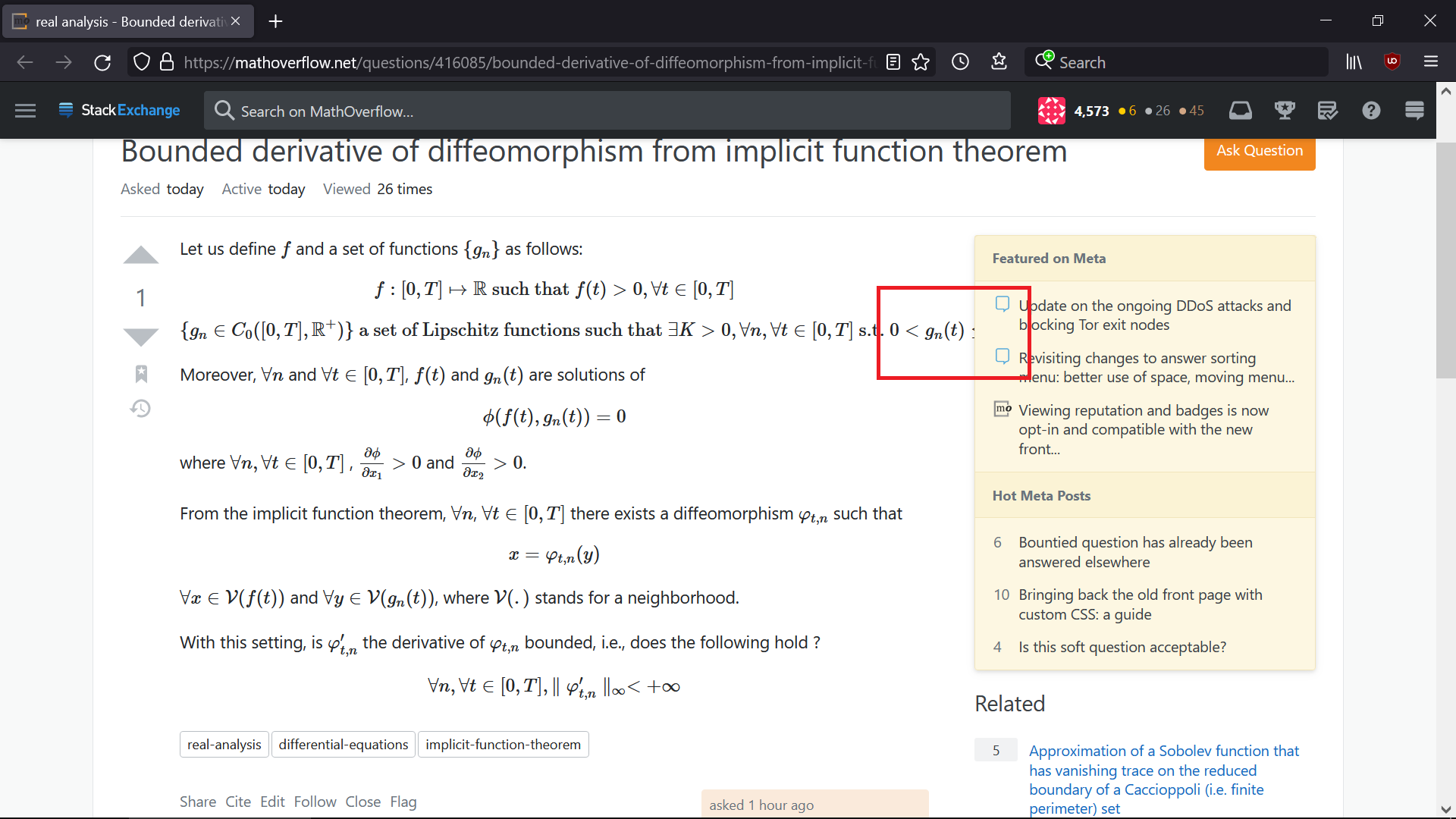
Let us define $f$ and a set of functions $\{g_n\}$ as follows: $$f:[0,T]\mapsto\mathbb{R}\textrm{ s.t. } f(t)>0,\forall t \in[0,T]$$ $$\{g_n:\mathbb{R}\mapsto\mathbb{R}^+\}\textrm{ a set of Lipschitz functions such that }\exists K>0,\forall n, \forall t\in[0,T] \textrm{ s.t. } 0 < g_n(t) \leq K <+\infty $$

$$\lim\limits_{n\to\infty} x_n = a \Longleftrightarrow (\forall U\in \mathcal O_a)(\exists n_0)(n\ge n_0 \Rightarrow x_n\in U) \Longleftrightarrow (\forall \varepsilon>0)(\exists n_0)(n\ge n_0 \Rightarrow |x_n-a|<\varepsilon)$$

$$ \begin{vmatrix} 1 & 3 & 1 & 2 \\ 2 & 3 & 0 & 1 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 1 & 3 & 1 & 2 \\ 1 & 0 &-1 &-1 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 0 & 3 & 0 &-1 \\ 0 & 0 &-2 &-4 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 0 & 3 & 0 &-1 \\ 0 & 0 &-2 &-4 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 &-1 &-2 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 0 & 1 \\ 0 & 2 & 0 &-1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 0 & 1 \\ 0 & 0 & 0 &-1 \\ \end{vmatrix}= 2\begin{vmatrix} 1 & 0 & 0 & 1 \\ 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 0 & 0 & 0 &-1 \\ \end{vmatrix}=2 $$

---

For comparison, this is the same text if we use inline formulas rather than centered formulas.

Let us define $f$ and a set of functions $\{g_n\}$ as follows: $f:[0,T]\mapsto\mathbb{R}\textrm{ s.t. } f(t)>0,\forall t \in[0,T]$, $\{g_n:\mathbb{R}\mapsto\mathbb{R}^+\}\textrm{ a set of Lipschitz functions such that }\exists K>0,\forall n, \forall t\in[0,T] \textrm{ s.t. } 0 < g_n(t) \leq K <+\infty $

$\lim\limits_{n\to\infty} x_n = a \Longleftrightarrow (\forall U\in \mathcal O_a)(\exists n_0)(n\ge n_0 \Rightarrow x_n\in U) \Longleftrightarrow (\forall \varepsilon>0)(\exists n_0)(n\ge n_0 \Rightarrow |x_n-a|<\varepsilon)$

$ \begin{vmatrix} 1 & 3 & 1 & 2 \\ 2 & 3 & 0 & 1 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 1 & 3 & 1 & 2 \\ 1 & 0 &-1 &-1 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 0 & 3 & 0 &-1 \\ 0 & 0 &-2 &-4 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= \begin{vmatrix} 0 & 3 & 0 &-1 \\ 0 & 0 &-2 &-4 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 &-1 &-2 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 1 & 3 \\ 0 & 2 & 1 & 1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 0 & 1 \\ 0 & 2 & 0 &-1 \\ \end{vmatrix}= 2\begin{vmatrix} 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 1 & 0 & 0 & 1 \\ 0 & 0 & 0 &-1 \\ \end{vmatrix}= 2\begin{vmatrix} 1 & 0 & 0 & 1 \\ 0 & 1 & 0 & 0 \\ 0 & 0 &-1 &-2 \\ 0 & 0 & 0 &-1 \\ \end{vmatrix}=2 $